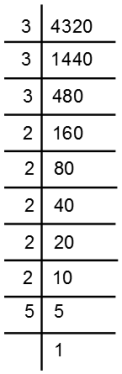
131. By what least number should 4320 be multiplied so as to obtain a number which a perfect cube ?
132. If x is a positive number, then which of the following fractions has the greatest value ?
133. By how much does $$\frac{6}{{7/8}}{\text{exceed }}\frac{{6/7}}{8} = ?$$
134. If $$\frac{4}{5}$$ of an estate be worth Rs. 16800, then the value of $$\frac{3}{7}$$ of the estate is = ?
135. $${\text{If }}\frac{{3a + 4b}}{{3c + 4d}}{\text{ = }}\frac{{3a - 4b}}{{3c - 4d}}{\text{ then}}$$
136. Simplify : $$\frac{{\frac{1}{3} + \frac{1}{4}\left[ {\frac{2}{5} - \frac{1}{2}} \right]}}{{1\frac{2}{3}\,{\text{of }}\frac{3}{4} - \frac{3}{4}\,{\text{of }}\frac{4}{5}}} = ?$$
137. $$\frac{{0.3555 \times 0.5555 \times 2.025}}{{0.225 \times 1.7775 \times 0.2222}}$$ is equal to = ?
138. $$\frac{1}{{10}}$$ of a pole is coloured red, $$\frac{1}{{20}}$$ white, $$\frac{1}{{30}}$$ blue, $$\frac{1}{{40}}$$ black, $$\frac{1}{{50}}$$ violet, $$\frac{1}{{60}}$$ yellow and the rest is green. If the length of the green portion of the pole is 12.08 metres, then the length of the pole is = ?
139. A drum of kerosene is $$\frac{3}{4}$$ full. When 30 liters of kerosene is drawn from it, it remains $$\frac{7}{{12}}$$ full. The capacity of the drums is = ?
140. A tree grows only $$\frac{3}{5}$$ as fast as the one beside it. In four years the combined growth of the trees is eight feet. How much does the shorter tree grow in 2 years?
Read More Section(Simplification)
Each Section contains maximum 100 MCQs question on Simplification. To get more questions visit other sections.