A rectangular plank $$\sqrt 2 $$ metre wide is placed symmetrically on the diagonal of a square of side 8 metres as shown in the figure. The area of the plank is :
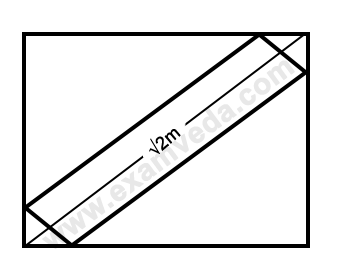
A. $$7\sqrt 2 $$ sq. m
B. 14 sq. m
C. 98 sq. m
D. ($$16\sqrt 2 $$ - 3) sq. m
Answer: Option B
Solution(By Examveda Team)
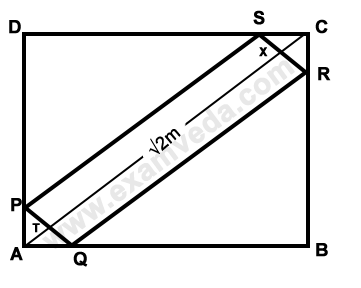
$$\eqalign{ & {\text{ Let }}AP{\text{ }} = {\text{ }}AQ{\text{ }} = {\text{ }}x{\text{ metres}} \cr & {\text{Then,}} \cr & \Rightarrow {x^2} + {x^2} = {\left( {\sqrt 2 } \right)^2} \cr & \Rightarrow 2{x^2} = 2 \cr & \Rightarrow {x^2} = 1 \cr & \Rightarrow x = 1\,m \cr & So,\,\,\vartriangle PAQ{\text{ is isosceles}}{\text{.}} \cr & \therefore PT = QT = \left( {\frac{{\sqrt 2 }}{2}} \right)m = \left( {\frac{1}{{\sqrt 2 }}} \right)m \cr & {\text{In }}\vartriangle {\text{ }}PTA{\text{, we have : }}\angle PTA = {90^ \circ } \cr & \therefore A{T^2} = A{P^2} - P{T^2} \cr & \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = {1^2} - {\left( {\frac{1}{{\sqrt 2 }}} \right)^2} \cr & \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 1 - \frac{1}{2} = \frac{1}{2} \cr & Or,AT = \left( {\frac{1}{{\sqrt 2 }}} \right)m \cr & {\text{Similarly,}} \cr & CX = \left( {\frac{1}{{\sqrt 2 }}} \right)m \cr & \therefore PS = QR = XT \cr & \,\,\,\,\,\,\,\,\,\,\,\,\,\, = AC - 2 \times AT \cr & \,\,\,\,\,\,\,\,\,\,\,\,\,\, = \left[ {8\sqrt 2 - \left( {2 \times \frac{1}{{\sqrt 2 }}} \right)} \right]m \cr & \,\,\,\,\,\,\,\,\,\,\,\,\,\, = \left( {8\sqrt 2 - \frac{2}{{\sqrt 2 }}} \right)m \cr & \,\,\,\,\,\,\,\,\,\,\,\,\,\, = \frac{{14}}{{\sqrt 2 }}m \cr & {\text{Area of the plank :}} \cr & = \left( {\frac{{14}}{{\sqrt 2 }} \times \sqrt 2 } \right){m^2} \cr & = 14\,{m^2} \cr} $$
Related Questions on Area
A. 15360 m2
B. 153600 m2
C. 30720 m2
D. 307200 m2
E. None of these
A. 2%
B. 2.02%
C. 4%
D. 4.04%
E. None of these
A. 16 cm
B. 18 cm
C. 24 cm
D. Data inadequate
E. None of these
The percentage increase in the area of a rectangle, if each of its sides is increased by 20% is:
A. 40%
B. 42%
C. 44%
D. 46%

Join The Discussion