From the foot and the top of a building of height 230 m, a person observes the top of a tower with angles of elevation of b and a respectively. What is the distance between the top of these buildings if tan a = $$\frac{5}{{12}}$$ and tan b = $$\frac{4}{{5}}$$

Solution(By Examveda Team)

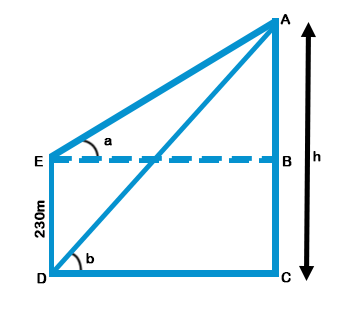
Let ED be the building and AC be the tower.
Given that ED = 230 m, ∠ADC = b, ∠AEB = a
Also given that tan a = $$\frac{5}{{12}}$$ and tan b = $$\frac{4}{{5}}$$
Let AC = h
Required Distance = Distance between the top of these buildings = AE
$$\eqalign{ & {\text{From}}\,{\text{the}}\,{\text{right}}\,\Delta ABE, \cr & \tan \left( a \right) = \frac{{AB}}{{BE}} \cr} $$
$$ \Rightarrow \frac{5}{{12}} = \frac{{\left( {h - 230} \right)}}{{BE}}$$     [∵ tan(a) = $$\frac{5}{{12}}$$ (given), AB = (AC - BC) = (AC - ED) = (h - 230)]
$$\eqalign{ & \Rightarrow BE = \frac{{12\left( {h - 230} \right)}}{5}\,.....\left( 1 \right) \cr & {\text{From}}\,{\text{the}}\,{\text{right}}\,\Delta ACD, \cr & \tan \left( b \right) = \frac{{AC}}{{CD}} \cr} $$
$$ \Rightarrow \frac{4}{5} = \frac{h}{{CD}}$$    [∵ tan(b) = $$\frac{4}{5}$$ (given), AC = h]
$$\eqalign{ & \Rightarrow CD = \frac{{5h}}{4}\,.....\left( 2 \right) \cr & {\text{From}}\,{\text{the}}\,{\text{diagram,}}\,BE = CD \cr} $$
$$ \Rightarrow \frac{{12\left( {h - 230} \right)}}{5} = \frac{{5h}}{4}$$     (from equation 1 & 2)
$$\eqalign{ & \Rightarrow 48h - \left( {4 \times 12 \times 230} \right) = 25h \cr & \Rightarrow 23h = \left( {4 \times 12 \times 230} \right) \cr & \Rightarrow h = \frac{{\left( {4 \times 12 \times 230} \right)}}{{23}} \cr & \,\,\,\,\,\,\,\,\,\,\,\,\, = 480\,{\text{m}}\,......\left( 3 \right) \cr & AB = \left( {AC - BC} \right) \cr} $$
$$\,\,\,\,\,\,\,\,\,\,\,\, = \left( {480 - 230} \right)$$    [∵ Since AC = h = 480 (from 3) and BC = ED = 230 m(given)]
$$\,\,\,\,\,\,\,\,\,\,\,\, = 250\,{\text{m}}$$
In the triangle ABE, tan(a) =$$\frac{5}{{12}}$$ . Let's figure out the value of sin(a) now.
Consider a triangle with opposite side = 5 and adjacent side = 12 such that tan(a) = $$\frac{5}{{12}}$$
$$\eqalign{ & {\text{hypotenuse}} = \sqrt {{5^2} + {{12}^2}} = 13 \cr & {\text{i}}{\text{.e}}{\text{.,}} \cr & \sin \left( a \right) = \frac{{{\text{opposite}}\,{\text{side}}}}{{{\text{hypotenuse}}}} = \frac{5}{{13}} \cr} $$
We have seen that $$\sin \left( a \right) = \frac{5}{{13}}$$
$$\eqalign{ & \Rightarrow \frac{{AB}}{{AE}} = \frac{5}{{13}} \cr & \Rightarrow AE = AB \times \frac{{13}}{5} \cr & = 250 \times \frac{{13}}{5} \cr & = 650\,{\text{m}} \cr} $$

i.e., Distance between the top of the buildings = 650 m