The ratio of the length of a rod and its shadow is 1 : $$\sqrt 3 $$ The angle of elevation of the sum is

A. 30°

B. 45°

C. 60°

D. 90°

Answer: Option A

Solution(By Examveda Team)

Let AB be rod and BC be its shadow
So that AB : BC = 1 : $$\sqrt 3 $$
Let $$\theta $$ be the angle of elevation

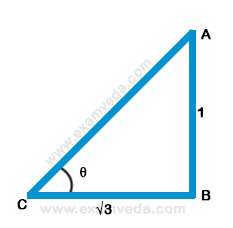
$$\eqalign{ & \therefore \tan \theta = \frac{{AB}}{{BC}} = \frac{1}{{\sqrt 3 }} = \tan {30^ \circ } \cr & \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\left( {\because \tan {{30}^ \circ } = \frac{1}{{\sqrt 3 }}} \right) \cr & \therefore \theta = {30^ \circ } \cr} $$
∴ Hence angle of elevation $$ = {30^ \circ }$$