Tree top’s angle of elevation is 30° from a point on ground, 300m away the tree. When the tree grew up its angle of elevation became 60° from the same point. How much did the tree grow?

Solution(By Examveda Team)

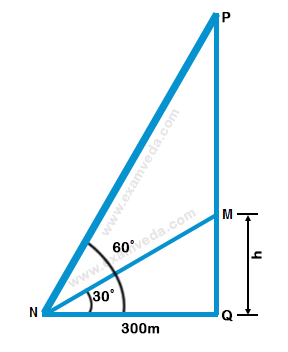
$$\eqalign{ & {\text{Original tree height}} = {\text{h}} = {\text{MQ}} \cr & {\text{New}}\,{\text{tree}}\,{\text{height}} = PQ \cr & {\text{in}}\,\Delta MQN,\,\tan {30^ \circ } = \frac{1}{{\sqrt 3 }} = \frac{{MQ}}{{NQ}} \cr & \therefore MQ = \frac{{300}}{{\sqrt 3 }} \cr & {\text{in}}\,\Delta PQN,\,\tan {60^ \circ } = \sqrt 3 = \frac{{PQ}}{{NQ}} \cr & \therefore PQ = 300\sqrt 3 \cr & {\text{Tree}}\,{\text{grew}} = PQ - MQ \cr & \therefore {\text{Tree}}\,{\text{grew}} \cr & = 300\sqrt 3 - \frac{{300}}{{\sqrt 3 }} \cr & = 300\frac{2}{{\sqrt 3 }} \cr & = 3 \times 100 \times \frac{2}{{\sqrt 3 }} \cr & = 200\sqrt 3 \,{\text{m}} \cr} $$